Cubes and Cube Roots

To understand cube roots, first you must understand cubes ...

How to Cube A Number

To cube a number, just use it in a multiplication 3 times ...

Example: What is 3 Cubed?

3 Cubed

=

=

3 × 3 × 3

=

27

Note: we write down "3 Cubed" as 3³
(the little "³" means the number appears three times in multiplying)

Some More Cubes

4 cubed	=	43	=	4 × 4 × 4	=	64
5 cubed	=	53	=	5 × 5 × 5	=	125
6 cubed	=	63	=	6 × 6 × 6	=	216

Cube Root

A cube root goes the other direction:

3 cubed is 27, so the cube root of 27 is 3

3

27

The cube root of a number is ...
... the special value that when cubed gives the original number.

The cube root of 27 is ...
... 3, because when 3 is cubed you get 27.

Note: When you see "root" think "I know the tree, but what is the root that produced it?" In this case the tree is "27", and the cube root is "3".

Here are some more cubes and cube roots:

4		64
5		125
6		216

Example: What is the Cube root of 125?

Well, we just happen to know that 125 = 5 × 5 × 5 (if you use 5 three times in a multiplication you will get 125) ...

... so the answer is 5

The Cube Root Symbol

This is the special symbol that means "cube root", it is the "radical"symbol (used for square roots) with a little three to mean cube root.

You can use it like this: 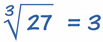 (you would say "the cube root of 27 equals 3")

You Can Also Cube Negative Numbers

Have a look at this:

If you cube 5 you get 125:		5 × 5 × 5 = 125
If you cube -5 you get -125:		-5 × -5 × -5 = -125

So the cube root of -125 is -5

Perfect Cubes

The Perfect Cubes are the cubes of the whole numbers:

	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	etc
Perfect Cubes:	1	8	27	64	125	216	343	512	729	1000	1331	1728	2197	2744	3375	...

It is easy to work out the cube root of a perfect cube, but it is really hard to work out other cube roots.

Example: what is the cube root of 30?

Well, 3 × 3 × 3 = 27 and 4 × 4 × 4 = 64, so we can guess the answer is between 3 and 4.

• Let's try 3.5: 3.5 × 3.5 × 3.5 = 42.875
• Let's try 3.2: 3.2 × 3.2 × 3.2 = 32.768
• Let's try 3.1: 3.1 × 3.1 × 3.1 = 29.791

We are getting closer, but very slowly ... at this point, I get out my calculator and it says:

3.1072325059538588668776624275224

... but the digits just go on and on, without any pattern. So even the calculator's answer is only anapproximation ! 

(Further reading: these kind of numbers are called surds which are a special type of irrational number)